The Coriolis Effect
Perhaps you’ve heard that your toilet water spins clockwise or counterclockwise depending on whether you live in the Northern or Southern Hemisphere.
This phenomenon is supposedly the result of the Coriolis effect.
This next sentence might be the oddest I’ve ever typed: Unfortunately, your hemispherical location does not influence your toilet, but the Coriolis effect is a real fictitious force.
Named for Gaspard-Gustave de Coriolis (fantastic name), the Coriolis force is known scientifically as a fictitious force. This term is a bit of a misnomer, as the Coriolis effect that is produced by the Coriolis force is very real. A fictitious force is one that appears to act on a mass whose motion is described in a non-inertial frame of reference. The key takeaway from this definition is the part about inertial frames of reference. This article could become very long if we examined the ideas with sufficient rigor, so we’ll try to stick to the explain-like-I’m-five versions. An inertial frame of reference is one in which an object is not being acted upon by net external forces and moves in a straight line. Think of science classes where the student learns about Newton’s laws, such as inertia. An object in motion will remain in motion unless acted upon by another force. Fictitious forces arise when our frames are non-inertial, meaning the frames are either accelerating or spinning. The two most famous fictitious forces are the centrifugal and the Coriolis. As an example, imagine yourself driving at a constant speed in a car. Suddenly, the car turns. You feel a force pushing you sideways. In reality, no force has pushed you sideways; instead, your body’s inertia wanted to remain moving forward, but your frame of reference (the car) accelerated, causing you to feel a version of the centrifugal force.
As it turns out, we live on a massive non-inertial frame of reference!
The toilets might not connect to the Coriolis effect, but, if you’ve ever wondered why hurricanes spin and why they spin in different directions in the two hemispheres, our boy Gaspard-Gustave has you covered.
Before we get to the storms, let’s explore the effect in general.
Picture the Earth, roughly a sphere. Our planet spins on an axis, which extends through the sphere near the poles. We spin all the way around in one day.
Now picture a person standing 20 miles away from the North Pole and another person standing on the equator. Both people take 24 hours to rotate to the same spot anew. However, the person standing at the equator moves a lot more than the person near the pole. Whip out the trusty distance equation: D = R x T (distance = rate times time). Since the Time for both people is the same and their Distances are different, their Rates must also be different. So, the person at the equator is actually moving through space much more quickly than the person near the pole!
Though we do not feel the spin of the planet, the closer we move to the equator, the faster we’re moving.
To illustrate the next part, I’ll borrow a few graphics from the National Oceanic and Atmospheric Administration. Imagine you’re riding a train that’s moving 100 miles per hour. On a track next to you, another train is ahead of you, travelling at 50 miles per hour. You have a soccer ball and the other train has a goal. As your train nears the slower one, you ready yourself for the opportune moment. Just as the goal is in line with your ball, you unleash a perfectly straight shot. Instead of a goal, however, your shoot zooms wide right. What happened? You forgot you would be imparting 100 MPH worth of sideways velocity on the ball. Though you kicked it straight, it missed the goal, which was only moving sideways at 50 miles per hour.
Now, let’s spread out our trains a bit.
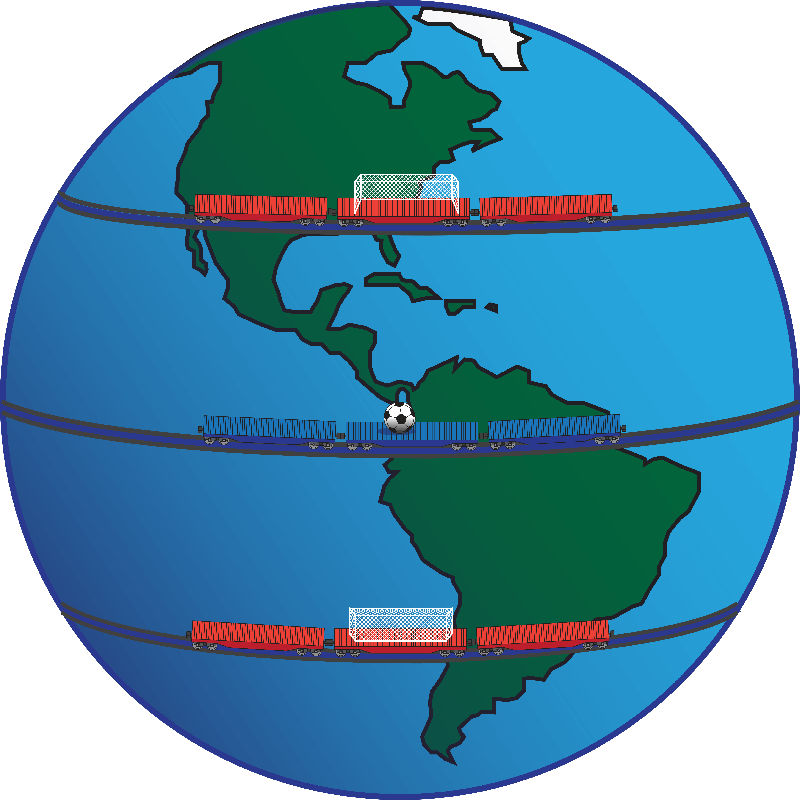
Imagine we’re on a train on the equator and two trains run parallel to us on the Tropic of Cancer and the Tropic of Capricorn. For this part, it might help you visualize if you imagine the trains to be stationary, though they can be moving at the same speed, too. If the trains are stationary, we know the train at the equator is still moving faster than the others because it has farther to go. However, from space, the trains would all appear to be moving the same speed!
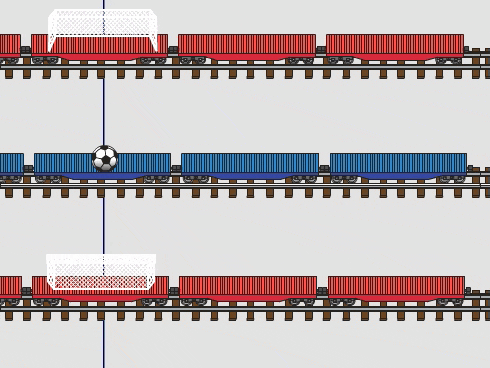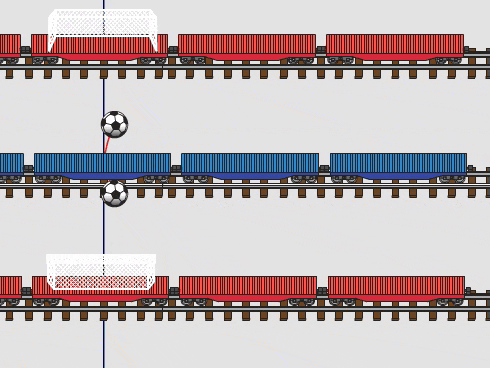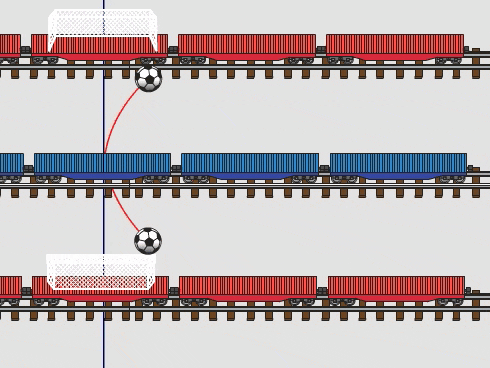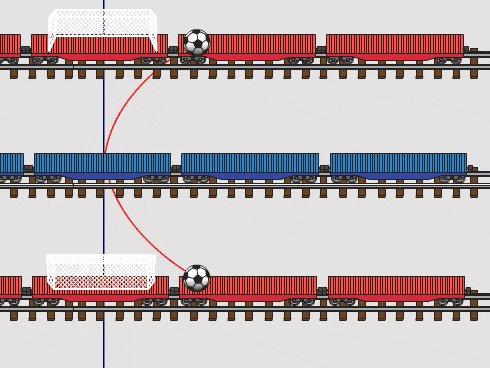
What happens if we attempt the world’s longest penalty kick?
The result is the same as the previous attempt!
The ball misses both goals by “curving” in different directions. This image is the Coriolis effect in basic form.
What is going on? Why does the ball miss? When the ball exits the train on the equator, it does so with a specific velocity vector. As it travels north or south, it maintains its east-west velocity. But, as it gets farther and farther from the equator, the ground beneath it is spinning more slowly. So, as it reaches the latitude of the goal, the ball is moving far more quickly than the net. From the sky, the ball would have “bent” or “deflected” away from the goal!
This phenomenon happens because of the Earth’s spinning, non-inertial frame of reference.
And though our soccer example was a metaphor, the effect great influences wind, water, and clouds.
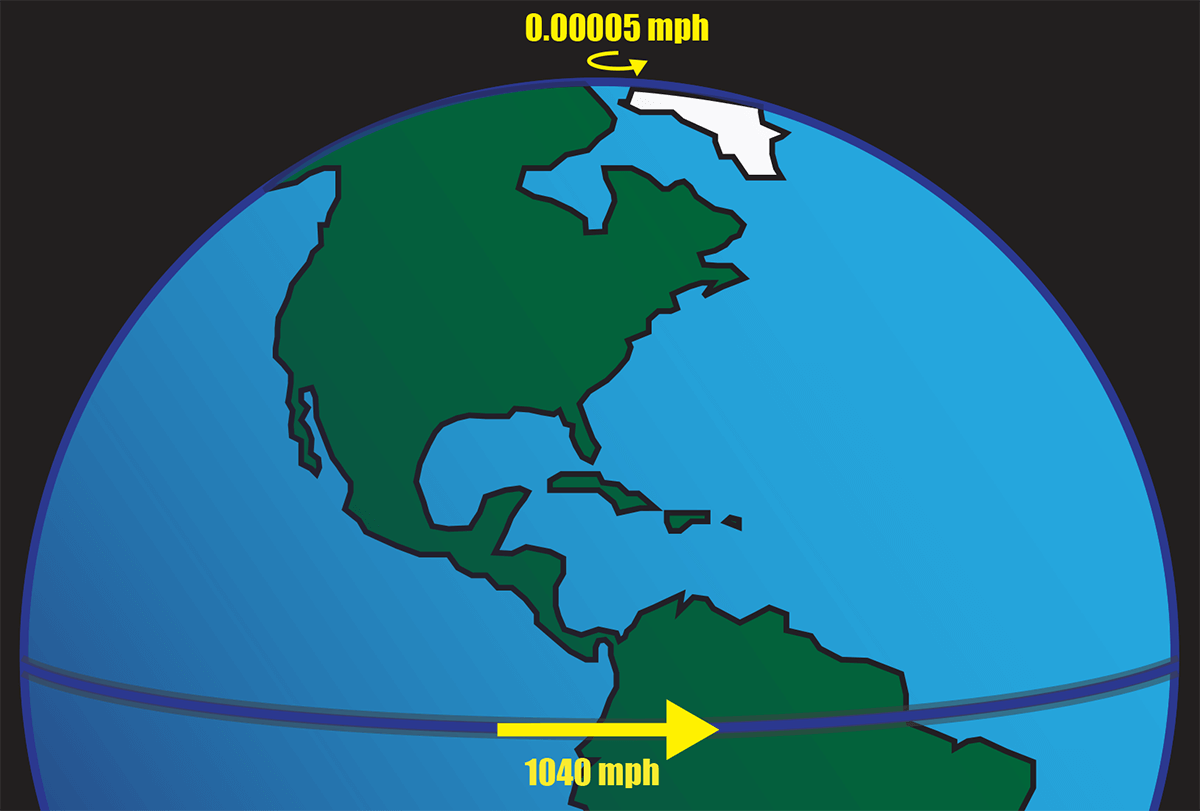
If a cloud forms on the equator, it will move at approximately 1,040 miles per hour. If the cloud moves north, it will maintain this velocity thanks to inertia, but everything it encounters will be moving more slowly. The result is a cloud that seemingly bends to the right (in the Northern Hemisphere), “outracing” all the clouds that formed on that latitude. Flip hemispheres and the cloud would “bend” to its left.
The same thing happens in reverse. If a cloud forms at the Tropic of Cancer and moves south, it will be moving more slowly than every other cloud it encounters. This original cloud will seemingly bend to its right (which would look like moving to the west from a celestial perspective) and “fall behidn” all its cloud buddies. Once again, the opposite happens from the Tropic of Capricorn heading north.
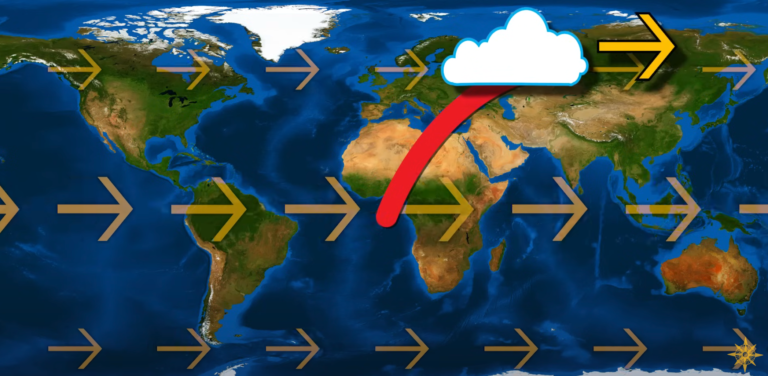
All the large-scale water and air constituents are subject to the Coriolis effect. Not just clouds, but winds and ocean waters. The effect gives us water currents and trade winds.
Clouds bending, thanks to relative speeds and frame of reference, makes sense, but how does the Coriolis effect cause a hurricane to spin?
For this portion, we need to introduce some basic meteorology. Most weather phenomena transpire thanks to the interplay between areas of high pressures and low pressures. Air moves from areas of high pressure toward areas of low pressure. The eye of a hurricane is an example of an area of extremely low pressure.
Since the eye is a tight circle, air from all directions is sucked toward it. In the Northern Hemisphere, air and clouds sucked toward the eye from the south will be traveling faster than the eye and will bend to the right. Air traveling from the north, will also bend toward the right from its perspective (west) because it will be traveling more slowly than the eye. The result of constant right bends around a stable eye is a hurricane that spins counterclockwise!
In the Southern Hemisphere, everything bends to the left, which causes a clockwise cyclone.
If the Coriolis effect truly shapes the direction of wind and water, why is it not responsible for the directions our toilets swirl?
In short, the answer is scale. The Coriolis effect meaningfully unfolds only over large distances. At global scales, we can see clouds bend and hurricanes twist. At toilet scale, though, the differences between velocities from north to south are not great enough to cause a water direction. To go back to the soccer analogy, if a field is aligned north-to-south, the distance between the penalty spot and the goal is not enough to influence the ball, even though the goal and the ball are traveling at minutely different speeds.
Instead, our toilet flows tend to be caused by the design of the bowls and the input of water.
So, no go on the toilet, but makes giant spinning storms of death. Pretty good for a fictitious force.
